త్రిభుజ సంఖ్య

మొదటి ఆరు త్రిభుజ సంఖ్యలు

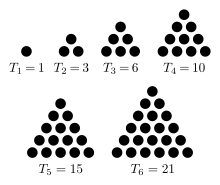
త్రిభుజ సంఖ్య అనగా ఒక సమబాహు త్రిభుజం యేర్పరచుటకు కావలసిన వస్తువుల సంఖ్య. "1" అను సంఖ్య త్రిభుజ సంఖ్య. రెండు వస్తువులు భుజంగా గల త్రిభుజం యేర్పరచాలంటే మూడు వస్తువులు కావాలి. అందువలన "3" త్రిభుజ సంఖ్య. మూడు వస్తువులు భుజంగా గల సమబాహు త్రిభుజం యేర్పరచాలంటే ఆరు వస్తువులు కావాలి. అందువలన "6" త్రిభుజ సంఖ్య అవుతుంది. అదేవిధంగా "n" వస్తువులు గల సమబాహు త్రిభుజం కావాలంటే "n", దాని తర్వాత సంఖ్య "n+1" లబ్దంలో సగ భాగము త్రిభుజ సంఖ్య అవుతుంది. పటంలో వివిధ త్రిభుజ సంఖ్యలను చూపడం జరిగినది.
కొన్ని త్రిభుజ సంఖ్యలను దిగువనీయబడినవి:

0, 1, 3, 6, 10, 15, 21, 28, 36, 45, 55, ....

త్రిభుజ సంఖ్యకు సూత్రము

త్రిభుజ సంఖ్యను ఈ క్రింది సూత్రం ద్వారా గణన చేయవచ్చు.

$T_{n}=\sum _{k=1}^{n}k=1+2+3+\dotsb +n={\frac {n(n+1)}{2}}={n+1 \choose 2}$

where $\textstyle {n+1 \choose 2}$ is a binomial coefficient. It represents the number of distinct pairs that can be selected from n + 1 objects, and it is read aloud as "n plus one choose two".

The triangular number T_n solves the handshake problem of counting the number of handshakes if each person in a room full of n + 1 total people shakes hands once with each other person. In other words, the solution to the handshake problem of n people is T_n-1.

Triangle numbers are the additive analog of the factorials, which are the products of integers from 1 to n.

The number of line segments between closest pairs of dots in the triangle can be represented with the following recurrence relation:

$L_{n}=L_{n-1}+3(n-1)$

In the limit, the ratio between the two numbers, dots and line segments is

$\lim _{n\to \infty }{\frac {T_{n}}{L_{n}}}={\frac {1}{3}}$